Essential Guide to Understanding Three Mechanical Waves Diagrams

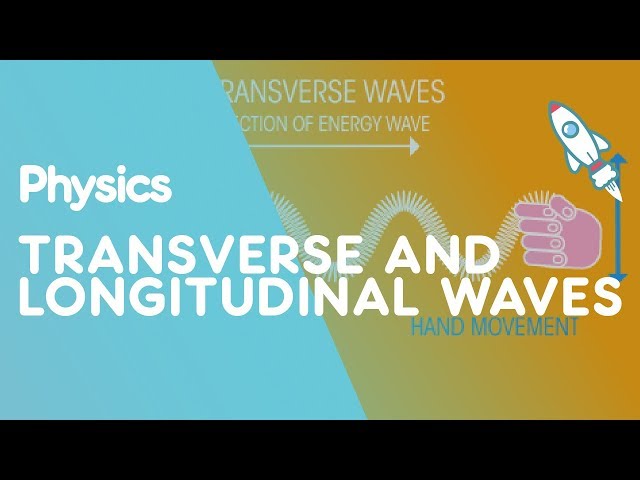
Three Mechanical Waves Diagram

A mechanical wave is a wave that propagates through a medium, such as water, air, or a solid. Mechanical waves are caused by a disturbance in the medium, such as a vibrating object or a moving fluid. The disturbance creates a wave that travels through the medium, causing the particles in the medium to oscillate.

There are three main types of mechanical waves: longitudinal waves, transverse waves, and surface waves. Longitudinal waves are waves in which the particles in the medium oscillate parallel to the direction of the wave’s propagation. Transverse waves are waves in which the particles in the medium oscillate perpendicular to the direction of the wave’s propagation. Surface waves are waves that travel along the boundary between two media, such as the surface of a liquid or the interface between two solids.

Here are some examples of charts and diagrams that can be used to represent three mechanical waves:

• Waveform diagram: A waveform diagram shows the displacement of a particle in the medium as a function of time. The waveform of a mechanical wave can be used to determine the wave’s amplitude, frequency, and wavelength.
• Ray diagram: A ray diagram shows the direction of propagation of a wave. The rays in a ray diagram are perpendicular to the wavefronts.
• Wavefront diagram: A wavefront diagram shows the surface of constant phase for a wave. The wavefronts of a mechanical wave are the surfaces on which the particles in the medium are oscillating in phase.

These charts and diagrams can be used to visualize the propagation of mechanical waves and to understand the properties of different types of waves.

Benefits of using charts and diagrams to represent mechanical waves:

• Charts and diagrams can help to visualize the propagation of mechanical waves.
• Charts and diagrams can be used to understand the properties of different types of waves.
• Charts and diagrams can be used to solve problems involving mechanical waves.

Tips for creating charts and diagrams of mechanical waves:

1. Choose the type of chart or diagram that is most appropriate for the purpose.
2. Use clear and concise labels.
3. Make sure that the chart or diagram is accurate.
4. Use a consistent scale.
5. Include a legend if necessary.

By following these tips, you can create charts and diagrams that are effective and informative.

Three Mechanical Waves Diagram

A mechanical wave diagram is a visual representation of the propagation of a mechanical wave. It can be used to show the wave’s amplitude, frequency, wavelength, and direction of propagation. Three key aspects of a mechanical wave diagram are:

• Waveform: The shape of the wave.
• Amplitude: The height of the wave.
• Wavelength: The distance between two consecutive crests or troughs.
• Frequency: The number of waves that pass a given point in one second.
• Direction of propagation: The direction in which the wave is traveling.
• Medium: The material through which the wave is traveling.
• Source: The object that creates the wave.
• Observer: The object that detects the wave.

These aspects are all interconnected and essential for understanding the behavior of mechanical waves. For example, the waveform of a wave is determined by its amplitude and wavelength. The frequency of a wave is determined by its wavelength and the speed of the wave. The direction of propagation of a wave is determined by the medium through which the wave is traveling. By understanding these relationships, we can use mechanical wave diagrams to analyze and predict the behavior of waves in a variety of situations.

Waveform

The waveform of a wave is the shape of the wave as it propagates through a medium. It is a graphical representation of the displacement of the medium as a function of time or position. The waveform of a mechanical wave can be used to determine the wave’s amplitude, frequency, and wavelength.

• Sine wave: A sine wave is a smooth, periodic wave that has a sinusoidal waveform. Sine waves are common in nature and can be found in sound waves, light waves, and water waves.
• Square wave: A square wave is a periodic wave that has a square waveform. Square waves are often used in electronics and digital.
• Triangle wave: A triangle wave is a periodic wave that has a triangular waveform. Triangle waves are often used in music synthesis.
• Sawtooth wave: A sawtooth wave is a periodic wave that has a sawtooth waveform. Sawtooth waves are often used in synthesizers and other electronic instruments.

The waveform of a mechanical wave is determined by the wave’s source and the medium through which the wave is traveling. For example, a sine wave is produced by a simple harmonic oscillator, while a square wave is produced by a digital oscillator. The medium through which the wave is traveling can also affect the waveform. For example, a sound wave traveling through air will have a different waveform than a sound wave traveling through water.

The waveform of a mechanical wave can be used to analyze the wave’s properties and behavior. For example, the amplitude of a wave can be determined by measuring the height of the wave’s peaks. The frequency of a wave can be determined by measuring the time between two consecutive peaks. The wavelength of a wave can be determined by measuring the distance between two consecutive peaks.Waveforms are an important tool for understanding the behavior of mechanical waves. They can be used to analyze the properties of waves, to identify different types of waves, and to solve problems involving waves.

Amplitude

In the context of a three mechanical waves diagram, the amplitude of each wave is represented by the height of its peaks and troughs. The amplitude is an important property of a wave, as it determines the wave’s energy and intensity. A wave with a large amplitude will have more energy and be more intense than a wave with a small amplitude.

• Relationship between amplitude and energy: The energy of a wave is proportional to the square of its amplitude. This means that a wave with a large amplitude will have more energy than a wave with a small amplitude.
• Relationship between amplitude and intensity: The intensity of a wave is proportional to the square of its amplitude. This means that a wave with a large amplitude will be more intense than a wave with a small amplitude.
• Amplitude and the human ear: The human ear is able to detect a wide range of amplitudes. The softest sounds that we can hear have an amplitude of about 10^-12 meters, while the loudest sounds that we can hear have an amplitude of about 1 meter.
• Amplitude and the human eye: The human eye is able to detect a wide range of amplitudes. The dimmest light that we can see has an amplitude of about 10^-10 meters, while the brightest light that we can see has an amplitude of about 1 meter.

The amplitude of a mechanical wave is an important property that can be used to understand the wave’s energy, intensity, and other properties. When analyzing a three mechanical waves diagram, it is important to pay attention to the amplitude of each wave in order to understand its behavior and effects.

Wavelength

In a three mechanical waves diagram, the wavelength of each wave is represented by the distance between two consecutive crests or troughs. The wavelength is an important property of a wave, as it determines the wave’s speed and frequency. A wave with a long wavelength will travel more slowly and have a lower frequency than a wave with a short wavelength.

The wavelength of a wave is inversely proportional to its frequency. This means that a wave with a long wavelength will have a low frequency, and a wave with a short wavelength will have a high frequency.

The wavelength of a wave is also affected by the medium through which the wave is traveling. A wave will travel more slowly in a denser medium, and will therefore have a shorter wavelength. Conversely, a wave will travel more quickly in a less dense medium, and will therefore have a longer wavelength.

The wavelength of a mechanical wave is an important property that can be used to understand the wave’s speed, frequency, and other properties. When analyzing a three mechanical waves diagram, it is important to pay attention to the wavelength of each wave in order to understand its behavior and effects.

Here are some examples of how the wavelength of a mechanical wave can be used in practice:

• Ocean waves: The wavelength of ocean waves can be used to predict the speed of the waves and the height of the waves.
• Sound waves: The wavelength of sound waves can be used to determine the pitch of the sound.
• Light waves: The wavelength of light waves can be used to determine the color of the light.

By understanding the connection between wavelength and the other properties of a mechanical wave, we can use three mechanical waves diagrams to analyze and predict the behavior of waves in a variety of situations.

Frequency

In a three mechanical waves diagram, the frequency of each wave is represented by the number of waves that pass a given point in one second. The frequency of a wave is an important property, as it determines the wave’s speed, wavelength, and energy. A wave with a high frequency will travel more quickly, have a shorter wavelength, and have more energy than a wave with a low frequency.

The frequency of a wave is inversely proportional to its wavelength. This means that a wave with a high frequency will have a short wavelength, and a wave with a low frequency will have a long wavelength. The frequency of a wave is also affected by the medium through which the wave is traveling. A wave will travel more slowly in a denser medium, and will therefore have a lower frequency. Conversely, a wave will travel more quickly in a less dense medium, and will therefore have a higher frequency.

The frequency of a mechanical wave is an important property that can be used to understand the wave’s speed, wavelength, and other properties. When analyzing a three mechanical waves diagram, it is important to pay attention to the frequency of each wave in order to understand its behavior and effects.

Here are some examples of how the frequency of a mechanical wave can be used in practice:

• Ocean waves: The frequency of ocean waves can be used to predict the speed of the waves and the height of the waves.
• Sound waves: The frequency of sound waves can be used to determine the pitch of the sound.
• Light waves: The frequency of light waves can be used to determine the color of the light.

By understanding the connection between frequency and the other properties of a mechanical wave, we can use three mechanical waves diagrams to analyze and predict the behavior of waves in a variety of situations.

Direction of propagation

In a three mechanical waves diagram, the direction of propagation of each wave is represented by the direction of the wavefronts. The direction of propagation is an important property of a wave, as it determines the direction in which the wave will travel and the objects that will be affected by the wave. For example, a wave that is traveling in the x-direction will affect objects that are located in the x-direction. The direction of propagation is also important for understanding the behavior of waves in complex environments, such as when waves are reflected or refracted.

The direction of propagation of a wave is determined by the source of the wave and the medium through which the wave is traveling. For example, a sound wave that is produced by a speaker will travel in the direction away from the speaker. A water wave that is produced by a boat will travel in the direction away from the boat. The direction of propagation can also be changed by objects that are in the path of the wave. For example, a wave that is reflected off of a wall will travel in the opposite direction.

The direction of propagation is an important property of a wave that can be used to understand the wave’s behavior and effects. When analyzing a three mechanical waves diagram, it is important to pay attention to the direction of propagation of each wave in order to understand its behavior and effects.

Medium

The medium is an important component of a three mechanical waves diagram because it determines the properties of the waves. The speed, wavelength, and amplitude of a wave are all affected by the medium through which it is traveling. For example, sound waves travel faster through water than they do through air, and light waves travel faster through glass than they do through air.

In a three mechanical waves diagram, the medium is often represented by a line or a region of space. The waves are shown traveling through the medium, and the properties of the waves are indicated by the shape and size of the waves. The diagram can be used to visualize the propagation of waves and to understand the effects of the medium on the waves.

The medium is an important consideration in many practical applications of waves. For example, the speed of sound waves is important in designing buildings and other structures to withstand earthquakes. The wavelength of light waves is important in designing optical instruments such as telescopes and microscopes. By understanding the effects of the medium on waves, we can design systems that use waves to achieve specific goals.

Source

In a three mechanical waves diagram, the source of each wave is represented by a point or a small region of space. The source is the object that creates the wave, and it determines the properties of the wave, such as its amplitude, frequency, and wavelength. The source can be a vibrating object, such as a loudspeaker or a guitar string, or it can be a moving object, such as a boat or a car.

• Facet 1: The Source as a Vibrating Object

  When a vibrating object is used as a source, the wave that is created is a periodic wave. The period of the wave is the same as the period of vibration of the object. The amplitude of the wave is proportional to the amplitude of vibration of the object. The frequency of the wave is the same as the frequency of vibration of the object.

• Facet 2: The Source as a Moving Object

  When a moving object is used as a source, the wave that is created is a non-periodic wave. The shape of the wave depends on the speed and direction of motion of the object. The amplitude of the wave is proportional to the speed of the object. The frequency of the wave is proportional to the speed of the object and the wavelength of the wave.

• Facet 3: Multiple Sources

  A three mechanical waves diagram can also be used to represent the propagation of waves from multiple sources. In this case, the diagram will show the superposition of the waves from each source. The resulting wave pattern will be more complex than the wave pattern from a single source.

• Facet 4: Applications

  Three mechanical waves diagrams are used in a variety of applications, such as acoustics, optics, and seismology. In acoustics, three mechanical waves diagrams are used to design concert halls and other spaces to improve the sound quality. In optics, three mechanical waves diagrams are used to design lenses and other optical components. In seismology, three mechanical waves diagrams are used to study the propagation of seismic waves through the Earth.

By understanding the relationship between the source of a wave and the properties of the wave, we can use three mechanical waves diagrams to analyze and predict the behavior of waves in a variety of situations.

Observer

In a three mechanical waves diagram, the observer is the object that detects the wave. The observer can be a person, an animal, or a machine. The observer’s position and orientation relative to the wave will affect the way that the wave is detected.

• Facet 1: The Observer’s Position

  The observer’s position relative to the wave will affect the amplitude and frequency of the wave that is detected. For example, an observer who is close to the source of the wave will detect a wave with a higher amplitude than an observer who is far away from the source of the wave. Similarly, an observer who is moving towards the source of the wave will detect a wave with a higher frequency than an observer who is moving away from the source of the wave.

• Facet 2: The Observer’s Orientation

  The observer’s orientation relative to the wave will affect the direction of the wave that is detected. For example, an observer who is facing the source of the wave will detect a wave that is traveling towards them. An observer who is facing away from the source of the wave will detect a wave that is traveling away from them.

• Facet 3: The Observer’s Sensitivity

  The observer’s sensitivity will affect the threshold at which the wave is detected. For example, an observer with a high sensitivity will be able to detect waves with a lower amplitude than an observer with a low sensitivity.

• Facet 4: Multiple Observers

  A three mechanical waves diagram can also be used to represent the detection of waves by multiple observers. In this case, the diagram will show the superposition of the waves that are detected by each observer. The resulting wave pattern will be more complex than the wave pattern from a single observer.

By understanding the relationship between the observer and the wave, we can use three mechanical waves diagrams to analyze and predict the behavior of waves in a variety of situations.

A mechanical wave diagram is a visual representation of the propagation of three mechanical waves. It shows the displacement of the medium as a function of time and position. Mechanical waves are waves that propagate through a medium, such as water, air, or a solid. They are caused by a disturbance in the medium, such as a vibrating object or a moving fluid. The disturbance creates a wave that travels through the medium, causing the particles in the medium to oscillate.

Mechanical wave diagrams are important because they allow us to visualize the propagation of waves and to understand the properties of different types of waves. For example, we can use mechanical wave diagrams to determine the wavelength, frequency, and amplitude of a wave. We can also use mechanical wave diagrams to understand the effects of different media on the propagation of waves.

Mechanical wave diagrams have a long history, dating back to the early days of physics. They were first used by scientists to study the propagation of sound waves. Today, mechanical wave diagrams are used in a wide variety of fields, including acoustics, optics, and seismology.

FAQs about Three Mechanical Waves Diagrams

Three mechanical waves diagrams are a useful tool for visualizing and understanding the propagation of mechanical waves. Here are some frequently asked questions about three mechanical waves diagrams:

Question 1: What is a mechanical wave?

Answer: A mechanical wave is a wave that propagates through a medium, such as water, air, or a solid. Mechanical waves are caused by a disturbance in the medium, such as a vibrating object or a moving fluid. The disturbance creates a wave that travels through the medium, causing the particles in the medium to oscillate.

Question 2: What is a three mechanical waves diagram?

Answer: A three mechanical waves diagram is a visual representation of the propagation of three mechanical waves. It shows the displacement of the medium as a function of time and position.

Question 3: What are the different types of mechanical waves?

Answer: There are three main types of mechanical waves: longitudinal waves, transverse waves, and surface waves. Longitudinal waves are waves in which the particles in the medium oscillate parallel to the direction of the wave’s propagation. Transverse waves are waves in which the particles in the medium oscillate perpendicular to the direction of the wave’s propagation. Surface waves are waves that travel along the boundary between two media, such as the surface of a liquid or the interface between two solids.

Question 4: What are the properties of a mechanical wave?

Answer: The properties of a mechanical wave include its wavelength, frequency, and amplitude. The wavelength is the distance between two consecutive crests or troughs of the wave. The frequency is the number of waves that pass a given point in one second. The amplitude is the height of the wave from its resting position.

Question 5: How are three mechanical waves diagrams used?

Answer: Three mechanical waves diagrams are used to visualize and understand the propagation of mechanical waves. They can be used to determine the wavelength, frequency, and amplitude of a wave. They can also be used to understand the effects of different media on the propagation of waves.

Question 6: What are the benefits of using three mechanical waves diagrams?

Answer: Three mechanical waves diagrams are a useful tool for visualizing and understanding the propagation of mechanical waves. They are simple to create and can be used to illustrate a variety of wave phenomena. They are also a valuable tool for teaching and learning about waves.

Summary: Three mechanical waves diagrams are a useful tool for visualizing and understanding the propagation of mechanical waves. They can be used to determine the wavelength, frequency, and amplitude of a wave. They can also be used to understand the effects of different media on the propagation of waves. Three mechanical waves diagrams are a valuable tool for teaching and learning about waves.

Next: Applications of Three Mechanical Waves Diagrams

Conclusion

In this article, we have explored the concept of three mechanical waves diagrams. We have learned about the different types of mechanical waves, the properties of mechanical waves, and the uses of three mechanical waves diagrams. Three mechanical waves diagrams are powerful tools for visualizing and understanding the propagation of mechanical waves. They can be used to solve a variety of problems in acoustics, optics, and seismology.

As we continue to learn more about the propagation of mechanical waves, we will be able to develop new technologies that use waves to improve our lives. For example, we may be able to develop new medical imaging techniques that use sound waves to visualize the inside of the body. We may also be able to develop new energy technologies that use waves to generate electricity. The possibilities are endless.

Youtube Video: